Pairs Trading Basics: Correlation, Co-integration And Strategy

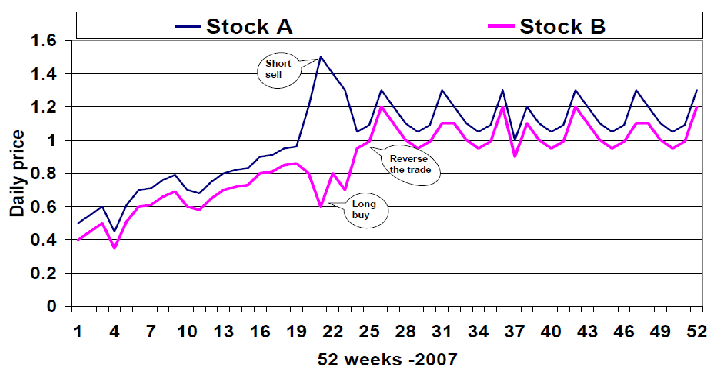
Pair trading is apparently quite possibly the most famous sorts of exchanging system. In this technique, generally a couple of stocks are exchanged a market-impartial system, for example it doesn't make a difference whether the market is moving upwards or downwards, the two vacant situations for each stock fence against one another. The vital difficulties two by two exchanging are to:
Pick a couple which will give you great factual exchange openings after some time

Pick the passage/leave focuses

Measurements assume an essential part in the principal challenge of choosing the pair to exchange. The pair is generally looked over similar bin of stocks, for example, Microsoft and Google (innovation space) or ICICI and Axis (Indian Banking) or Nifty Index and MSCI record (market files). Among every area, there are a huge number of sets are conceivable. The best ones are those which depend on numerical or factual tests. We will find out around two measurable strategies in the following part of sets exchanging.

Relationship

Despite the fact that not normal, a couple of Pairs Trading techniques see connection to locate a reasonable pair to exchange.

Connection is measured by the relationship coefficient ρ, which goes from - 1 to +1. The relationship coefficient shows the level of connection between's the two factors. The estimation of +1 implies there exists an ideal positive relationship between's the two factors, - 1 methods there is an ideal negative connection and 0 methods there is no relationship.

An ideal positive connection is the point at which one variable moves either up or down way, the other variable likewise moves a similar way with a similar size while an ideal negative relationship is the point at which one variable moves the upward way, the other variable moves in the descending (for example inverse) course with a similar size.

The relationship coefficient for the two factors is given by
Correlation(X,Y) = ρ = COV(X,Y)/SD(X).SD(Y)
where, cov (X, Y) is the covariance between X and Y while SD (X) and SD(Y) indicates the standard deviation of the individual factors.

In the event that the connection is high, say 0.8, dealers may pick that pair for sets exchanging. This high number addresses a solid connection between the two stocks. So if A goes up, the odds of B going up are likewise very high. In light of this supposition a market unbiased procedure is played where An is purchased and B is sold; purchased and sold choices are made dependent on their individual examples, structured products.

Simply seeing relationship may give you misleading outcomes. For example, if your sets exchanging methodology depends on the spread between the costs of the two stocks, it is conceivable that the costs of the two stocks continue expanding without ever mean-returning.

Spread = log(a) – nlog(b), where 'a' and 'b' are costs of stocks An and B separately.
For each load of A got, you have sold n supplies of B.
Presently, both 'a' and 'b' increments so that the estimation of spread abatements. This will bring about a misfortune since stock An is expanding at a rate lower than stock B and you are lacking in stock B.
Along these lines, one ought to be cautious about utilizing just relationship for sets exchanging.
Allow us presently to move to the following area two by two exchanging rudiments, ie Cointegration.

Coi-ntegration

The most widely recognized test for Pairs Trading is the cointegration test. Cointegration is a factual property of at least two time-arrangement factors which demonstrates if a straight blend of the factors is fixed.
Allow us to comprehend this assertion above. The double cross arrangement factors, for this situation, are the log of costs of stocks An and B. Direct mix of these factors can be a straight condition characterizing the spread:
As you probably are aware, Spread = log(a) – nlog(b), where 'a' and 'b' are costs of stocks An and B separately.
For each load of A got, you have sold n supplies of B.

In the event that An and B are cointegrated, at that point it infers that this condition above is fixed. A fixed cycle has entirely significant highlights which are needed to demonstrate Pairs Trading procedures. For example, for this situation, if the condition above is fixed, that proposes that the mean and fluctuation of this condition stays consistent over the long haul. So on the off chance that we start with 'n', which is known as the fence proportion, so that spread = 0, the property of fixed suggests that the normal estimation of spread will stay as 0. Any deviation from this normal worth is a case for factual anomaly, henceforth a case for sets exchanging!
In view of the hypothesis, let us attempt to respond to the inquiry which you may be considering, in the following segment of Pairs exchanging essentials.